- Chapter 2 chapter exercises, exercise 15, p. 79:
An insect population (denoted by y) is being destroyed by an
insecticide at a constant rate d. It is increasing at a rate
proportional to the current population; the constant of
proportionality is g. Which of the following initial-value
problems might be a reasonable model of this situation? Justify
your rejection of each unacceptable choice.
- (a)

- (b)
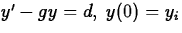
- (c)
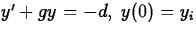
- (d)
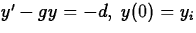
- Section 5.1, exercise 7, p.217: The text derived
the model
for the forced, undamped vertical spring-mass system. It also considered a spring-mass system without forcing that was damped by friction. It obtained the model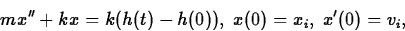
In fact, even a freely suspended mass is subject to damping due to air resistance and internal resistance in the spring. These damping forces can be modeled as being proportional to velocity and acting in the opposite direction, just like the force of friction acting on the mass sliding horizontally.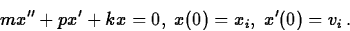
- (a)
- Compare the undamped, forced model with the damped model
without forcing. Predict what a model of a forced, damped
system would look like.
- (b)
- Verify your prediction by deriving such a model.
Specifically, consider the system shown in figure 5.5 and
suppose that the mass is subject to air resistance which is
proportional to its velocity and acts in the opposite
direction.